SPHERICAL MIRRORS
A mirror whose polished, reflecting surface is a part of a hollow sphere of glass or plastic is called a spherical mirror. In a spherical mirror, one of the two curved surfaces is coated with a thin layer of silver followed by a coating or red lead oxide paint. Thus one side of the spherical mirror is opaque and the other side is a highly polished reflecting surface.
Depending on the nature of the reflecting surface of a mirror, the spherical mirror is classified into two types.
(1) Concave mirror
(2) Convex mirror
CONCAVE MIRROR
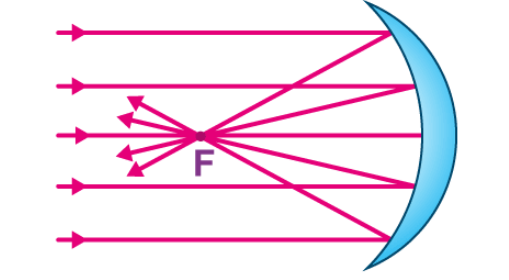
We know that a spherical mirror is a hole in a reflecting sphere. A concave mirror has a reflected surface that curves inward. A lot of people use concave mirrors as shaving mirrors, by dentists, and even in binoculars. The field of view is small, but the picture that is reflected is bigger. It's also possible to call concave mirrors merging mirrors.
CONVEX MIRROR
If the reflective surface is curved outward, it is classified as a convex mirror. Convex mirrors are often utilized in automotive mirrors. The focal length influences the size of the reflected image, resulting in a reduction in size while increasing the field of view. This is highly beneficial for drivers, as blind areas are significantly minimized. Convex mirrors are also referred to as fisheye or diverging mirrors. These mirrors are immediately identifiable due to their prominent outward protrusion. The Organization of Spherical Mirrors
Every larger virtual sphere always includes a spherical mirror. Imagine a line passing the center of this spherical touching the reflecting surface at its center. The main axis runs here.

A point A in the diagram marks the junction of the main axis with the reflecting surface. The vertex of the mirror, Point A, is its centre.
Point C in the picture marks the center of the sphere from whence the spherical mirror has been cut. This represents the curvature's centre. Denoted by the figure's length 'R', the radius of curvature is the distance from the vertex to the centre of curvature.
The focal point marked as "F" in the figure lies midway between the vertex and the center of curvature. The focal length of the mirror is the figure's "f" length measured from one end. It is the distance the mirror's focal point separates from the vertex.
Though the graphic above shows a concave mirror, the characteristics of convex mirrors also stay the same. Their variations lie in the way they reflect light rays. Parabolic reflectors are among the non-spherical mirrors also available. When and for which purposes do we apply various types of mirrors?
Uses of Concave Mirrors
Shaving Mirrors: Concave mirrors are often used in shaving mirrors because they magnify the reflection, allowing for a closer shave.
Makeup Mirrors: Similar to shaving mirrors, concave mirrors help in applying makeup by providing a magnified view of the face.
Flashlights: In flashlights, concave mirrors are used to focus light into a beam, enhancing brightness and directionality.
Telescopes: Concave mirrors are utilized in telescopes to gather and focus light from distant celestial objects, improving visibility.
Solar Cookers: Concave mirrors can concentrate sunlight onto a small area, generating heat for cooking purposes.
Projectors: In some types of projectors, concave mirrors are used to direct and focus light onto the projection surface.
Medical Equipment: Concave mirrors are used in dental and medical instruments to provide magnified images of small areas.
Optical Devices: Various optical devices, including microscopes, utilize concave mirrors to enhance image quality and brightness.
Uses of Convex Mirrors
Traffic Safety: Convex mirrors are commonly used at road intersections and curves to provide a wider field of view, helping drivers see oncoming traffic.
Security: These mirrors are often installed in stores and warehouses to monitor blind spots and prevent theft.
Parking Assistance: Convex mirrors are used in parking lots to help drivers see around corners and avoid collisions.
Surveillance: They are utilized in surveillance systems to cover large areas without requiring multiple cameras.
Rear-View Mirrors: Convex mirrors are used in vehicles as rear-view mirrors to provide a broader view of the area behind the car.
Safety in Elevators: Convex mirrors can be found in elevators to allow passengers to see if the area is clear before exiting.
Industrial Applications: In factories, convex mirrors help workers see around machinery and improve safety in busy environments.
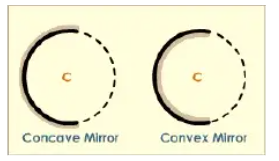
TERMS RELATED TO SPHERICAL MIRRORS Centre of Curvature: Center of curvature is centre of the sphere of which the spherical mirror forms a part. It is denoted by the letter C.
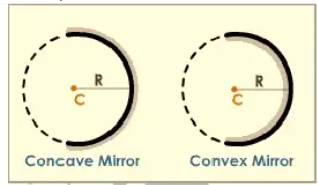
Radius of curvature: Radius of curvature is the radius of the sphere of which the mirror is a part. It is represented by the letter R.
Linear aperture: Linear aperture is the distance between the extreme points (X and Y) on the periphery of the mirror.
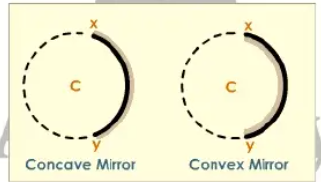
XY is the aperture
Pole: Pole is the midpoint of the aperture of the spherical mirror. It is represented by the letter P. Midpoint of XY= Pole (P).
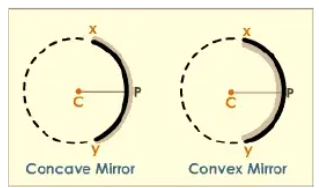
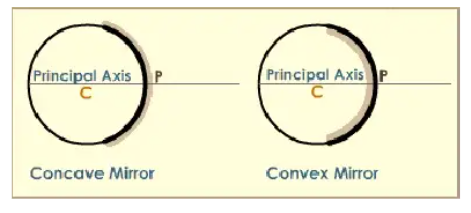
Principal Axis: Principal axis is the straight line passing through thepole and the center of curvature of a spherical mirror.
Principal Focus or Focus:
The principal focus of a spherical mirror be defined as a pointon its principal axis where a beam of light parallel to the principal axis converges to orappears to diverge from after reflection from the spherical mirror.

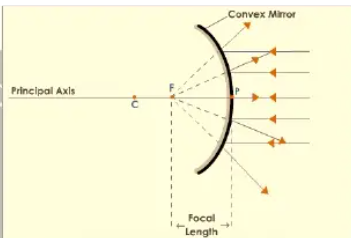
Focal Length:
Focal length is the distance between the pole and the focus of a mirror. Itis represented by the letter f.
RELATION BETWEEN FOCAL LENGTH AND RADIUS OF CURVATURE Concave Mirror
Setup:
Imagine a concave mirror with its pole at point P, center of curvature at point C, and focal point at point F.
Draw the principal axis (the line PC).
Consider a ray of light AB parallel to the principal axis, incident on the mirror at point B.
Reflection:
The ray AB is reflected by the mirror. According to the laws of reflection, the angle of incidence (∠ABC) equals the angle of reflection (∠CBF).
Draw the normal to the mirror at point B, which is the line BC (because the radius of a sphere is perpendicular to the tangent at any point on its surface). Geometric Relationships:
Let ∠ABC = ∠CBF = θ (theta).
Since AB is parallel to PC, ∠BCP = ∠ABC = θ (alternate interior angles).
Now, consider triangle BCF. Since ∠CBF = θ and ∠BCP = θ, triangle BCF is an isosceles triangle. Therefore, CF = FB.
Also, consider triangle BPC.
Small Aperture Approximation:
Here's where the small aperture assumption comes in. If the aperture of the mirror is small, point B is very close to point P.
Consequently, FB ≈ FP.
Deriving the Relationship:
Since CF = FB and FB ≈ FP, we can say CF ≈ FP.
We know that CP = R (radius of curvature).
Also, CP = CF + FP.
Substituting CF ≈ FP, we get CP ≈ FP + FP, or CP ≈ 2FP.
Since FP = f (focal length), we have R ≈ 2f.
Therefore, f ≈ R/2.
Therefore, in an ideal spherical mirror, f=R/2.
Convex Mirror Derivation (Conceptual Overview):
The convex mirror derivation follows a similar geometric approach, but with the following key differences:
The reflected rays diverge, creating a virtual image.
The focal point and center of curvature are behind the mirror.
The angles are measured differently due to the diverging nature of the reflected rays.
However, the same small angle approximations, and laws of reflection are used to arrive at the same final equation of f=R/2.
Comentários